Stretching a spring analysis (F=ke)
I can describe and calculate the properties of a spring using Hooke’s law.


Stretching a spring analysis (F=ke)
I can describe and calculate the properties of a spring using Hooke’s law.
Link copied to clipboard
These resources will be removed by end of Summer Term 2025.
Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
These resources were created for remote use during the pandemic and are not designed for classroom teaching.
Lesson details
Key learning points
- A spring is elastically deformed if it returns to its original shape after the applied force is removed
- A spring is inelastically deformed if it does not return to its original shape after the applied force is removed
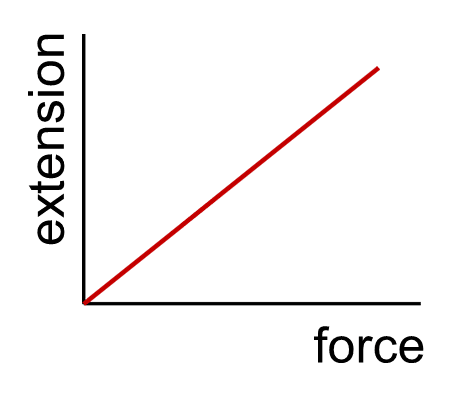
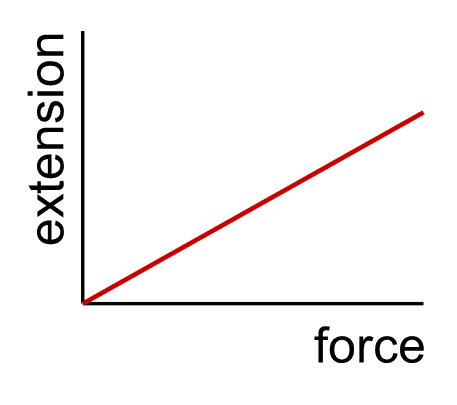
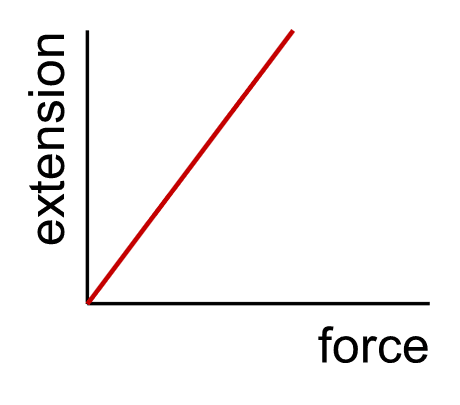
- Elastic deformation is proportional to the force applied to stretch or compress a spring
- The spring constant (k) is the force needed to extend or compress an elastic object by one metre
- Force on a spring = spring constant × extension
Keywords
Directly proportional - A variable is directly proportional to another if it doubles or triples when the other one does the same.
Elastic deformation - The stretch of a spring that returns to its original shape when the stretching force is removed.
Inelastic deformation - The stretch of a spring as it is permanently stretched out of shape.
Spring constant - A measure of how stiff a spring is and how hard it is to stretch or compress.
Hooke’s Law - For elastic deformations, extension (or compression) is directly proportional to the force applied.
Common misconception
Pupils often think the bigger the spring constant, the more a spring stretches for a given force.
Provide opportunities for pupils to apply understanding that the spring constant describes the stiffness of a spring. The bigger the spring constant, the harder it is to stretch (or compress) the spring.
To help you plan your year 10 combined science lesson on: Stretching a spring analysis (F=ke), download all teaching resources for free and adapt to suit your pupils' needs...
To help you plan your year 10 combined science lesson on: Stretching a spring analysis (F=ke), download all teaching resources for free and adapt to suit your pupils' needs.
The starter quiz will activate and check your pupils' prior knowledge, with versions available both with and without answers in PDF format.
We use learning cycles to break down learning into key concepts or ideas linked to the learning outcome. Each learning cycle features explanations with checks for understanding and practice tasks with feedback. All of this is found in our slide decks, ready for you to download and edit. The practice tasks are also available as printable worksheets and some lessons have additional materials with extra material you might need for teaching the lesson.
The assessment exit quiz will test your pupils' understanding of the key learning points.
Our video is a tool for planning, showing how other teachers might teach the lesson, offering helpful tips, modelled explanations and inspiration for your own delivery in the classroom. Plus, you can set it as homework or revision for pupils and keep their learning on track by sharing an online pupil version of this lesson.
Explore more key stage 4 combined science lessons from the Energy of moving objects unit, dive into the full secondary combined science curriculum, or learn more about lesson planning.

Licence
Starter quiz
6 Questions
Exit quiz
6 Questions