Myths about teaching can hold you back


- Year 9
Pythagoras' theorem in context
I can use and apply Pythagoras' theorem to solve problems in a range of contexts.


- Year 9
Pythagoras' theorem in context
I can use and apply Pythagoras' theorem to solve problems in a range of contexts.
These resources will be removed by end of Summer Term 2025.
Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
These resources were created for remote use during the pandemic and are not designed for classroom teaching.
Lesson details
Key learning points
- Within context, it can be difficult to initially identify whether Pythagoras' theorem can be used.
- Pythagoras' theorem may be used if a right-angled triangle can be drawn, even if drawn within a different shape.
- Pythagoras' theorem can be used to check for properties of other shapes involving right-angles.
Keywords
Perpendicular height - The perpendicular height is the perpendicular distance from the base to the opposite vertex.
Pythagoras’ theorem - Pythagoras’ theorem shows that the sum of the squares of the two shorter sides of a right-angled triangle is equal to the square of its longest side (the hypotenuse).
Hypotenuse - A hypotenuse is the side of the right-angled triangle which is opposite the right-angle.
Common misconception
I need to be given a triangle in order to use Pythagoras' theorem.
You do not need to be given a triangle. It is an important skill to be able to sketch and label a triangle for yourself from given information, or go straight into an algebraic stage of calculation if aware that lengths given are perpendicular.
To help you plan your year 9 maths lesson on: Pythagoras' theorem in context, download all teaching resources for free and adapt to suit your pupils' needs...
To help you plan your year 9 maths lesson on: Pythagoras' theorem in context, download all teaching resources for free and adapt to suit your pupils' needs.
The starter quiz will activate and check your pupils' prior knowledge, with versions available both with and without answers in PDF format.
We use learning cycles to break down learning into key concepts or ideas linked to the learning outcome. Each learning cycle features explanations with checks for understanding and practice tasks with feedback. All of this is found in our slide decks, ready for you to download and edit. The practice tasks are also available as printable worksheets and some lessons have additional materials with extra material you might need for teaching the lesson.
The assessment exit quiz will test your pupils' understanding of the key learning points.
Our video is a tool for planning, showing how other teachers might teach the lesson, offering helpful tips, modelled explanations and inspiration for your own delivery in the classroom. Plus, you can set it as homework or revision for pupils and keep their learning on track by sharing an online pupil version of this lesson.
Explore more key stage 3 maths lessons from the Geometrical properties: similarity and Pythagoras' theorem unit, dive into the full secondary maths curriculum, or learn more about lesson planning.

Equipment
Licence
Prior knowledge starter quiz
6 Questions
Q1.Izzy writes down a correct Pythagoras' theorem equation for a triangle. Which of the three sides of this triangle is the hypotenuse?
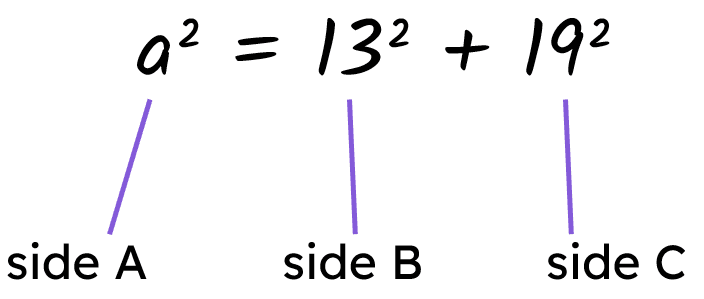
Q2.Aisha rearranges a correct Pythagoras' theorem equation for a triangle. Which of these statements are correct?
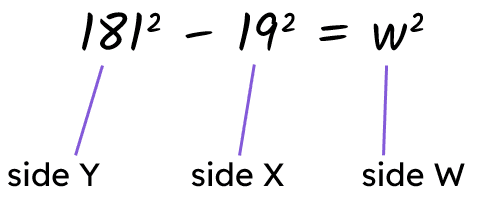
Q3.Which of these are correct for this triangle?
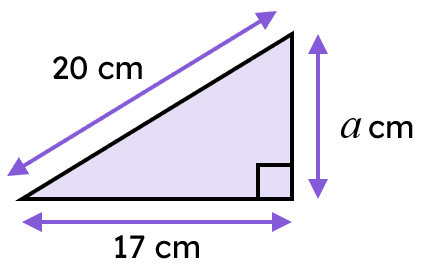
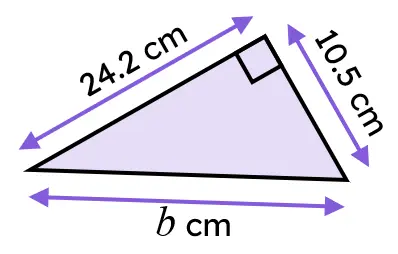
Q4.What is the value of $$b$$, rounded to 2 d.p.? $$b=$$ .
Q5.The diagonals of this kite have been drawn on. The value of $$x$$ is .
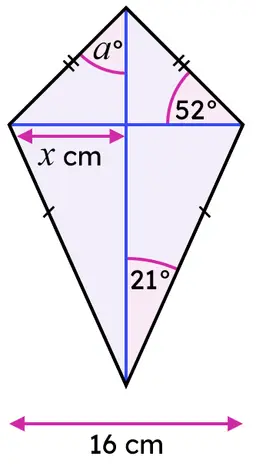
Q6.The diagonals of this kite have been drawn on. Which of these statements are correct.

Assessment exit quiz
6 Questions
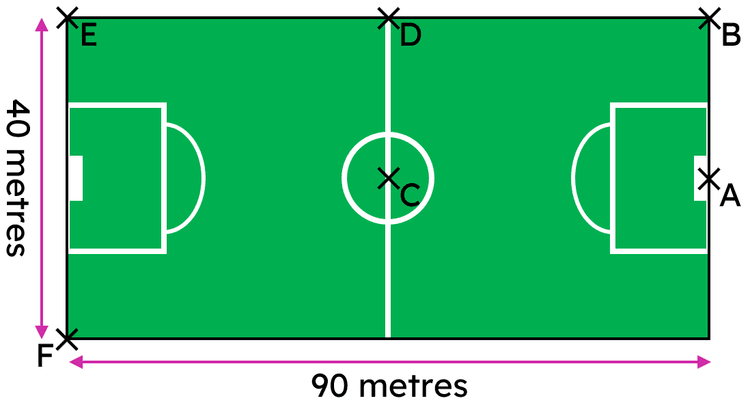
Q1.On this football pitch, the goalkeeper (at point A) wants to kick the ball to a striker (at point E), however the goalkeeper's longest kick is 91 m. Which of these statements are correct?
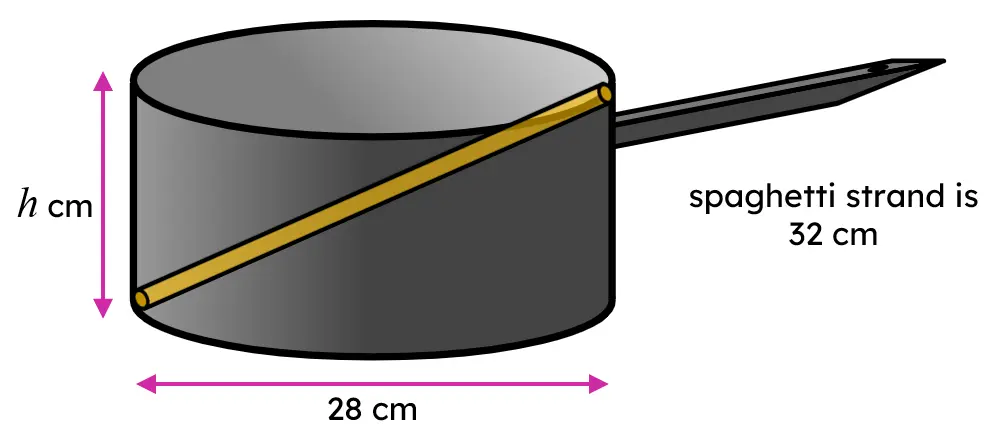
Q2.A factory produces cylindrical saucepans whose maximum base length is 28 cm. What must the minimum integer height of the saucepan be (in cm) so it can fully submerge a spaghetti noodle.
Q3.An archer fires an arrow that travels 20 metres to hit a target that is 9 m above ground. The arrow then drops to the ground. The archer must walk m (to 2 d.p.) to pick up the arrow.
Q4.An archer fires an arrow at a target 20 m away. The arrow drops 9 m to the ground. Jun uses Pythagoras' theorem to find how far the archer walks to collect the arrow. What assumptions does Jun make?
Q5.The captain (at C) passes the ball to the goalkeeper (at A), who then passes to the midfielder (at D), who then passes to a striker (at F). The total distance the ball travelled is m (to 2 d.p.).

Q6.Two bamboo sticks are placed on the diagonal of this kite to support it during high winds. The length of the vertical bamboo stick is cm (to 2 d.p.).


