Skip to content
Oak National Academy
Oak National Academy
Teachers
Pupils
New
New
Year 10
Foundation

Problem solving with similarity

I can use my knowledge of similarity to solve problems.

Download all resources
arrow-right
Share activities with pupils
arrow-right
New
New
Year 10
Foundation

Problem solving with similarity

I can use my knowledge of similarity to solve problems.

Download all resources
arrow-right
Share activities with pupils
arrow-right
warning

These resources will be removed by end of Summer Term 2025.

Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
View new resources
arrow-right

Lesson details

Key learning points

  1. A scaled version of a shape is very useful.
  2. Being able to scale the perimeter can reduce the number of calculations.
  3. Enlargement (transformations) and similar shapes are connected.

Keywords

  • Similar - Two shapes are similar if the only difference between them is their size. Their side lengths are in the same proportions.

  • Invariant - A property of a shape is invariant if that property has not changed after the shape is transformed.

  • Enlargement - Enlargement is a transformation that causes a change of size.

  • Scale factor - A scale factor is the multiplier between similar shapes that describes how large one shape is compared to the other.

Common misconception

A ratio of 1 : 400 for a scale model of a car to a real car will have units of cm and metres, because a scale model is measured in cm and a real car is measured in metres.

Unless the scale explicitly includes different units of measure for each part, you must always use the same unit for each part of a ratio. So, 1 : 400 should be seen as 1 cm : 400 cm, not 400 metres, which is far too long for a car!

Different maps and scale drawings will show their map scales and render sizes in different ways. This includes digital maps. It is important to understand what each value or ratio represents in each context.
Teacher tip

Licence

This content is © Oak National Academy Limited (2024), licensed on Open Government Licence version 3.0 except where otherwise stated. See Oak's terms & conditions (Collection 2).

Lesson video

Skip lesson video

Loading...

Starter quiz

Download starter quiz

6 Questions

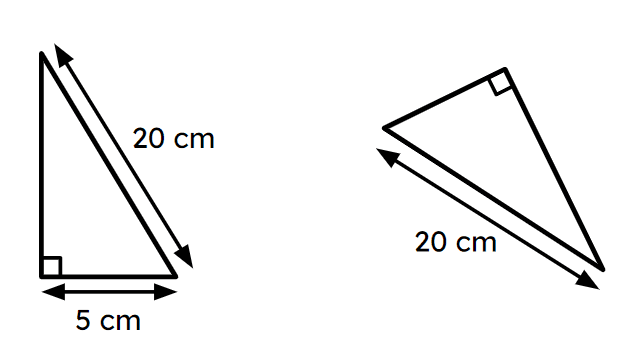
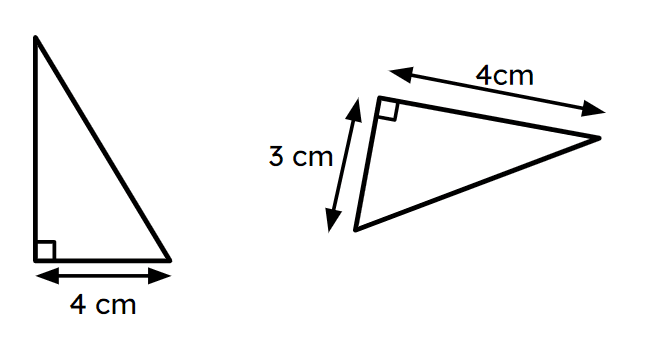
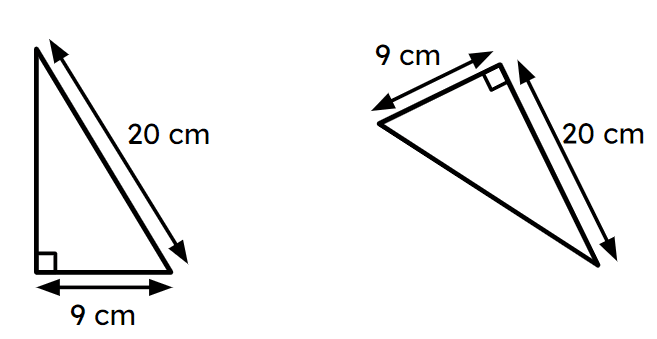
Q1.
Which of these pairs of triangles are congruent?
Correct Answer: An image in a quiz
An image in a quiz
An image in a quiz
An image in a quiz
An image in a quiz
Q2.
Pythagoras’ theorem states that the of the squares of the two shorter sides of a right-angled triangle is equal to the square of the hypotenuse.
Correct Answer: sum
Q3.
The lengths of the 3 edges of some triangles are given. Select all the right-angled triangles.
5 cm, 10 cm, 15 cm
Correct answer: 5 cm, 12 cm, 13 cm
12 cm, 28 cm, 24 cm
20 cm, 24 cm, 26 cm
Correct answer: 10 cm, 24 cm, 26 cm
Q4.
A right-angled triangle has a hypotenuse of 65 mm. Select the possible lengths of the two shorter sides.
Correct answer: 39 mm and 52 mm
20 mm and 35 mm
20 mm and 25 mm
Correct answer: 16 mm and 63 mm
32 cm and 40 cm
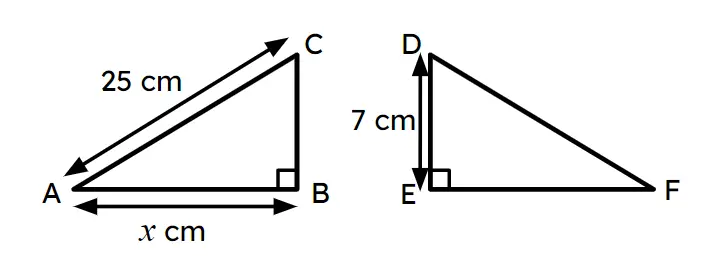
Q5.
Triangle ABC and triangle DEF are congruent and AB > BC. The length of the side $$x$$ is cm.
An image in a quiz
Correct Answer: 24
Q6.
Match each letter which the correct statement to complete the proof that triangle DAC and triangle ABC are congruent.
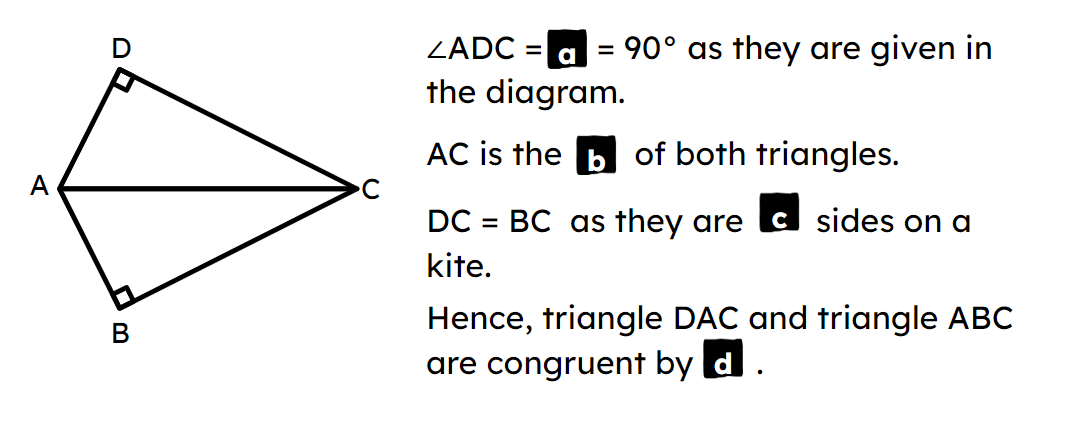
An image in a quiz
Correct Answer:a, ∠ABC

∠ABC

Correct Answer:b,hypotenuse

hypotenuse

Correct Answer:c,adjacent

adjacent

Correct Answer:d,RHS

RHS

Exit quiz

Download exit quiz

6 Questions

Q1.
Similarity can be used in architecture or in mechanical drawings to make a drawing of a real world object.
Correct Answer: scale
Q2.
This is a scale drawing of a van. The height of the van is metres.
An image in a quiz
Correct Answer: 2.4, 2.40, 2.4 m, 2.40 m
Q3.
This is a scale drawing of a van. The length of the van is metres.
An image in a quiz
Correct Answer: 4.8, 4.80, 4.8 m, 4.80 m
Q4.
Sam has attempted to enlarge this object by a scale factor of 2.5. Which statements are correct?
An image in a quiz
Sam is wrong, you can't have a decimal scale factor
Correct answer: The object and image aren’t similar because Sam enlarged it incorrectly.
Correct answer: The object and image aren't similar as the angles are not invariant
Object and image are similar as all enlargements are similar to their object.
The object and image are similar as the angles are invariant
Q5.
The dashed triangle shows the route a bird took between its nest N, a lake L and an old manor house M. The bird flies direct from its nest to the manor and then to the lake. How far does it fly?
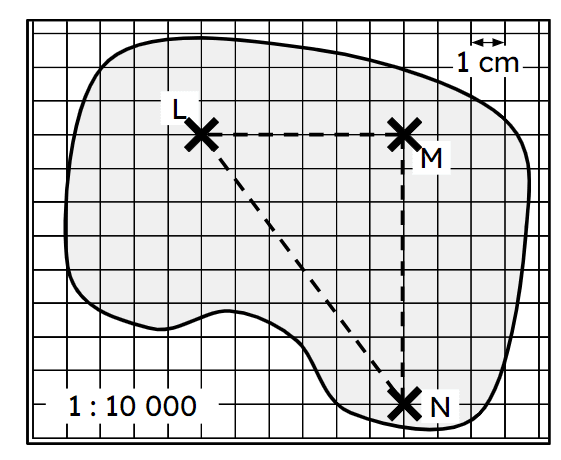
An image in a quiz
14 km
8 km
6 km
Correct answer: 1.4 km
0.2 km
Q6.
The dashed triangle shows the route a bird took between its nest N, a lake L and an old manor house M. The bird flies direct from its nest, N, to the lake, L. The distance the bird flies is km.
An image in a quiz
Correct Answer: 1, one