Myths about teaching can hold you back
- Year 11
- Higher
Checking and securing understanding of surface area of cuboids
I can efficiently calculate the surface area of cubes and cuboids.
- Year 11
- Higher
Checking and securing understanding of surface area of cuboids
I can efficiently calculate the surface area of cubes and cuboids.
These resources will be removed by end of Summer Term 2025.
Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
These resources were created for remote use during the pandemic and are not designed for classroom teaching.
Lesson details
Key learning points
- The surface area of a solid is the sum of the area of all the faces.
- The net of a cuboid can help find the surface area.
- The surface area of a cuboid can be found without drawing the net.
Keywords
Surface area - The surface area is the total area of all the surfaces of a closed 3D shape. The surfaces include all faces and any curved surfaces.
Net - The net of a 3D object is a 2D representation of its surfaces that can be folded up into the 3D object.
Common misconception
When working out the surface area, the pupil may only find the sum of the areas of three faces of a cuboid (for example the visible sides in a 3D drawing), instead of all six faces.
Encourage pupils to sketch the net of the cuboid as part of their solution. This will provide a visual reminder to the pupil to find the area of all six faces, as well as making it easier to check their answer.
To help you plan your year 11 maths lesson on: Checking and securing understanding of surface area of cuboids, download all teaching resources for free and adapt to suit your pupils' needs...
To help you plan your year 11 maths lesson on: Checking and securing understanding of surface area of cuboids, download all teaching resources for free and adapt to suit your pupils' needs.
The starter quiz will activate and check your pupils' prior knowledge, with versions available both with and without answers in PDF format.
We use learning cycles to break down learning into key concepts or ideas linked to the learning outcome. Each learning cycle features explanations with checks for understanding and practice tasks with feedback. All of this is found in our slide decks, ready for you to download and edit. The practice tasks are also available as printable worksheets and some lessons have additional materials with extra material you might need for teaching the lesson.
The assessment exit quiz will test your pupils' understanding of the key learning points.
Our video is a tool for planning, showing how other teachers might teach the lesson, offering helpful tips, modelled explanations and inspiration for your own delivery in the classroom. Plus, you can set it as homework or revision for pupils and keep their learning on track by sharing an online pupil version of this lesson.
Explore more key stage 4 maths lessons from the 2D and 3D shape: surface area and volume (pyramids, spheres and cones) unit, dive into the full secondary maths curriculum, or learn more about lesson planning.

Licence
Prior knowledge starter quiz
6 Questions
Q1.A prism is a __________ with a base that is a polygon and a parallel opposite face that is identical. The corresponding edges of the two polygons are joined by parallelograms.
Q2.Which of these 3D solids is a prism?



Q3.A set of cuboids each have a volume of 240 cm³. Match each base of a cuboid to the correct height.
Height: 10 cm
Height: 12 cm
Height: 4 cm
Height: 5 cm
Height: 6 cm
Q4.The volume of this prism is cm³.
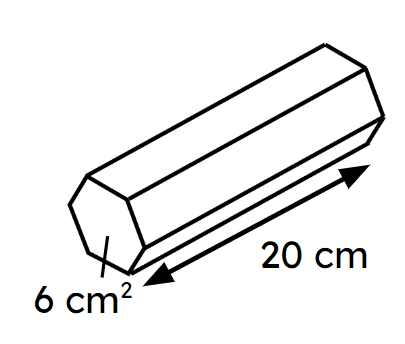
Q5.The volume of this triangular prism is cm³.

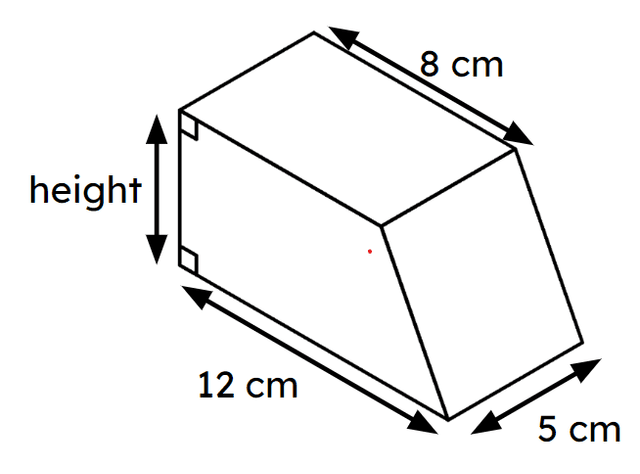
Q6.The volume of this prism is 280 cm³. The height of the prism is cm.
Assessment exit quiz
6 Questions
Q1.The surface area is the __________ area of all the surfaces of a closed 3D shape.
Q2.Here is a net of a cube. Which calculations correctly give the surface area of the cube?
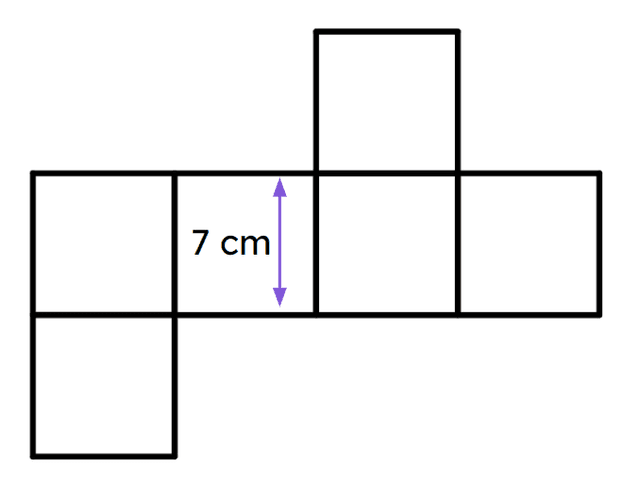
Q3.Here is a net of a cuboid. What is the surface area of the cuboid?

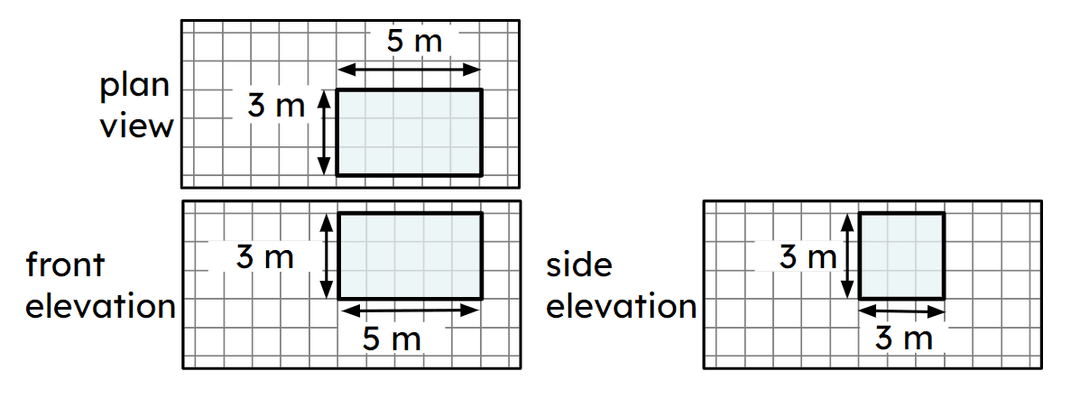
Q4.The diagram shows the plan view and front and side elevations of a cuboid. The surface area of the cuboid is m².
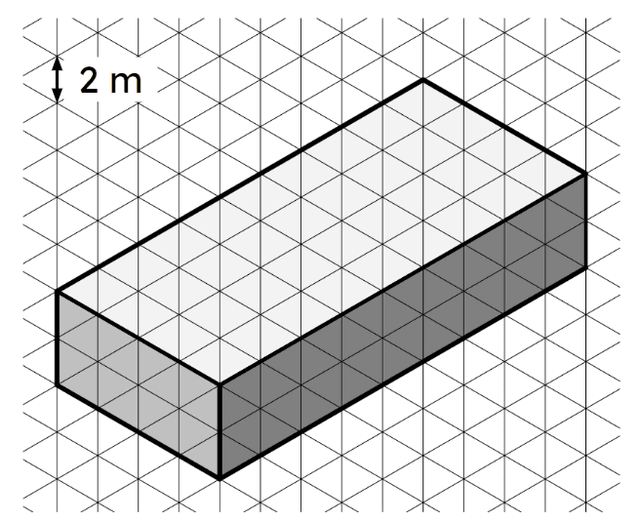
Q5.The diagram shows an isometric drawing of a cuboid. The surface area of the cuboid is m².