Skip to content
Oak National Academy
Oak National Academy
Teachers
Pupils
icon-background-square
New
New
Year 11
Higher

Algebra in tree and Venn diagrams

I can apply my knowledge of probability equations to tree and Venn diagrams to solve problems.

Download all resources
arrow-right
Share activities with pupils
arrow-right
icon-background-square
New
New
Year 11
Higher

Algebra in tree and Venn diagrams

I can apply my knowledge of probability equations to tree and Venn diagrams to solve problems.

Download all resources
arrow-right
Share activities with pupils
arrow-right
warning

These resources will be removed by end of Summer Term 2025.

Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
View new resources
arrow-right

Lesson details

Key learning points

  1. An equation can be constructed to find unknown probabilities in tree diagrams
  2. This equation can be manipulated and solved to find unknown probabilities in tree diagrams
  3. An equation can be constructed to find unknown probabilities in Venn diagrams
  4. This equation can be manipulated and solved to find unknown probabilities in Venn diagrams

Keywords

  • Probability - The probability that an event will occur is the proportion of times the event is expected to happen in a suitably large experiment.

  • Probability tree - Each branch of a probability tree shows a possible outcome from an event or from a stage of a trial, along with the probability of that outcome happening.

  • Independent events - Event A is independent of event B if the probability of event A occurring is not affected by whether or not event B occurs.

  • Mutually exclusive - Mutually exclusive events have no outcomes in common.

  • Venn diagram - Venn diagrams are a representation used to model statistical/probability questions. Commonly circles are used to represent events.

Common misconception

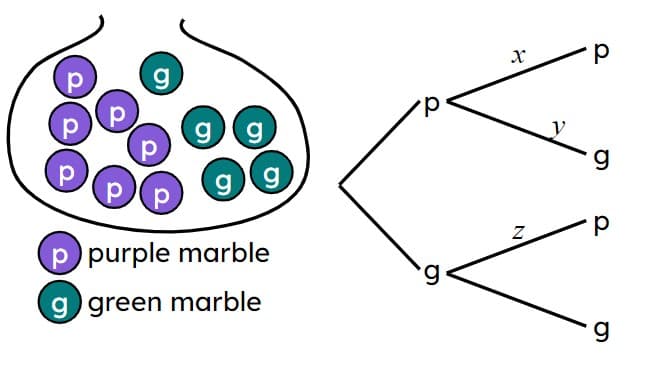
When variables are used in diagrams, pupils may be unsure whether it represents a probability, frequency or a particular outcomes.

Variables can be used to represent either of these pieces of information. It can be helpful to start a problem by writing down what the variables represent (e.g. \"Let x = the total number of marbles).


To help you plan your year 11 maths lesson on: Algebra in tree and Venn diagrams, download all teaching resources for free and adapt to suit your pupils' needs...

Pupils will find this lesson easier if they are already confident with manipulating algebraic fractions and solving quadratic equations.
speech-bubble
Teacher tip
copyright

Licence

This content is © Oak National Academy Limited (2025), licensed on Open Government Licence version 3.0 except where otherwise stated. See Oak's terms & conditions (Collection 2).

Lesson video

Skip lesson video

Loading...

Starter quiz

Download starter quiz

6 Questions

Q1.
Two events A and B are mutually exclusive. This means that....
Correct answer: P(A ∩ B) = 0
P(A ∪ B) = 0
P(A) + P(B) = 0
Correct answer: P(A | B) = 0
P(A | B') = 0
Q2.
Sofia has to catch a bus and then a train to get to school. When the bus is late, Sofia is more likely to miss the train and so be late for school. Match each value to its probability.
An image in a quiz
Correct Answer:$$x$$,0.05
tick

0.05

Correct Answer:$$y$$,0.2
tick

0.2

Correct Answer:$$z$$,0.9
tick

0.9

Q3.
Sofia has to catch a bus and then a train to get to school. When the bus is late, Sofia is more likely to miss the train and so be late for school. P(Sofia is late for school) = .
An image in a quiz
Correct Answer: 0.195
Q4.
Here is a general probability tree. Which of these probabilities give the probability of the highlighted events?
An image in a quiz
P(A' ∪ C')
Correct answer: P(A' ∩ C')
P(A') × P(C' | A)
Correct answer: P(A') × P(C' | A')
P(A') × P(A' | C')
Q5.
One marble is taken at random from a bag of 7 purple and 5 green marbles. The marble is not replaced. A second marble is then taken. The tree diagram shows these two events. Find the value of $$z$$.
An image in a quiz
$$7 \over 12$$
$$5 \over 12$$
Correct answer: $$7 \over 11$$
$$6 \over 11$$
$$5 \over 11$$
Q6.
One marble is taken at random from a bag of purple and green marbles. The marble is not replaced. A second marble is then taken. The tree diagram shows the two events. Find P(at least 1 green marble).
An image in a quiz
$$\frac{35}{132}$$
$$\frac{35}{132}$$
$$\frac{95}{144}$$
Correct answer: $$\frac{15}{22}$$
$$\frac{5}{33}$$

Exit quiz

Download exit quiz

6 Questions

Q1.
This probability Venn diagram shows the probability of a random spinner (spun once) landing on a square number or a factor of 36. Which of these probability statements are correct?
An image in a quiz
Correct answer: $$a + b + c + d = 1$$
P(square) = $$a$$
Correct answer: P(factor of 36) = $$b+c$$
P(square ∪ factor of 36) = $$b$$
P(square | factor of 36) = $$a$$
Q2.
This probability Venn diagram shows the probability of a random spinner (spun once) landing on a square number or a factor of 36. The value of $$x$$ is . Give your answer as a decimal.
An image in a quiz
Correct Answer: 0.1
Q3.
Here is a frequency Venn diagram. P(A ∩ B) = 0.2. The total frequency is .
An image in a quiz
Correct Answer: 15
Q4.
P(D given C) = 0.4. Which of these equations is correct to find the value of $$x$$?
An image in a quiz
Correct answer: $$\frac{2x}{3x+6}=0.4$$
$$\frac{2x}{x+6}=0.4$$
$$\frac{x+6}{2x}=0.4$$
$$\frac{x+6}{3x+6}=0.4$$
Q5.
A pack of cards contains 30 “character” (c) cards and some “spell” (s) cards. A card is taken at random, replaced and a 2nd card is taken. P(two spell cards) = 0.25. There are cards in the deck.
An image in a quiz
Correct Answer: 60
Q6.
The ratio of cats (c) to dogs (d) in an animal shelter is 4 : 3. Two random animals are taken from the shelter for adoption. Find an expression for P(2 cats).
An image in a quiz
$$\frac{4(4x-1)}{49x}$$
$$\frac{16}{49}$$
$$\frac{(4x-1)}{(7x-1)}$$
Correct answer: $$\frac{4(4x-1)}{7(7x-1)}$$